|
|
|
A number ![]() for which the Harmonic Mean of the Divisors of
for which the Harmonic Mean of the Divisors of ![]() , i.e.,
, i.e.,
![]() , is an
Integer, where
, is an
Integer, where ![]() is the number of Positive integral Divisors of
is the number of Positive integral Divisors of ![]() and
and ![]() is the
Divisor Function. For example, the divisors of
is the
Divisor Function. For example, the divisors of ![]() are 1, 2, 4, 5, 7, 10, 14, 20, 28, 35, 70, and 140, giving
are 1, 2, 4, 5, 7, 10, 14, 20, 28, 35, 70, and 140, giving
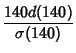 |
For distinct Primes ![]() and
and ![]() , harmonic divisor numbers are equivalent to Even Perfect Numbers for numbers of the form
, harmonic divisor numbers are equivalent to Even Perfect Numbers for numbers of the form ![]() . Mills (1972) proved that if there exists an Odd Positive harmonic divisor
number
. Mills (1972) proved that if there exists an Odd Positive harmonic divisor
number ![]() , then
, then ![]() has a prime-Power factor greater than
has a prime-Power factor greater than ![]() .
.
Another type of number called ``harmonic'' is the Harmonic Number.
See also Divisor Function, Harmonic Number
References
Edgar, H. M. W. ``Harmonic Numbers.'' Amer. Math. Monthly 99, 783-789, 1992.
Garcia, M. ``On Numbers with Integral Harmonic Mean.'' Amer. Math. Monthly 61, 89-96, 1954.
Guy, R. K. ``Almost Perfect, Quasi-Perfect, Pseudoperfect, Harmonic, Weird, Multiperfect and Hyperperfect Numbers.''
§B2 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 45-53, 1994.
Mills, W. H. ``On a Conjecture of Ore.'' Proceedings of the 1972 Number Theory Conference. University
of Colorado, Boulder, pp. 142-146, 1972.
Ore, Ø. ``On the Averages of the Divisors of a Number.'' Amer. Math. Monthly 55, 615-619, 1948.
Pomerance, C. ``On a Problem of Ore: Harmonic Numbers.'' Unpublished manuscript, 1973.
Sloane, N. J. A. Sequence
A007340/M4299
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and extended entry in
Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
Zachariou, A. and Zachariou, E. ``Perfect, Semi-Perfect and Ore Numbers.'' Bull. Soc. Math. Gréce (New Ser.)
13, 12-22, 1972.
|
|
|
© 1996-9 Eric W. Weisstein