|
|
|
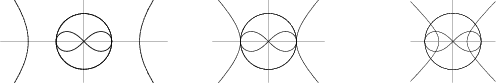
For a Hyperbola with ![]() with Inversion Center at the center, the Inverse Curve
with Inversion Center at the center, the Inverse Curve
![$\displaystyle {2k\cos t\over a[3-\cos(2t)]}$](h_1992.gif) |
(1) | ||
![$\displaystyle {k\sin(2t)\over a[3-\cos(2t)]}$](h_1993.gif) |
(2) |
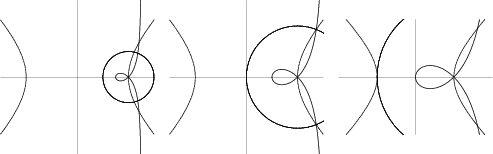
For an Inversion Center at the Vertex, the Inverse Curve
![$\displaystyle a+{4k\cos t\sin^2({\textstyle{1\over 2}}t)\over a[5-4\cos t+\cos(2t)-2\sin(2t)]}$](h_1995.gif) |
(3) | ||
![$\displaystyle a+{k(\tan t-1)\over a[(\sec t-1)^2+(\tan t-1)^2]}$](h_1996.gif) |
(4) |
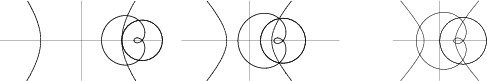
For an Inversion Center at the Focus, the Inverse Curve
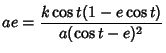 |
(5) | ||
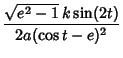 |
(6) |
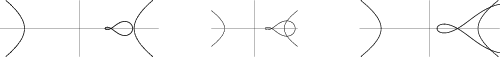
For a Hyperbola with ![]() and Inversion Center at the
Vertex, the Inverse Curve
and Inversion Center at the
Vertex, the Inverse Curve
![$\displaystyle b+{2k\cos t(\sqrt{3}-\cos t)\over b[9-4\sqrt{3}\cos t+\cos(2t)-2\sin(2t)]}$](h_2002.gif) |
(7) | ||
![$\displaystyle b+{k(\tan t-1)\over b[(\sqrt{3}\sec t-1)^2+(\tan t-1)^2]}$](h_2003.gif) |
(8) |
References
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, p. 203, 1972.