Koch Antisnowflake

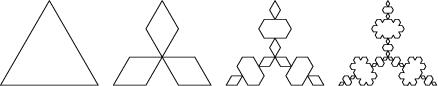
$\begin{figure}\begin{center}\BoxedEPSF{KochAntisnowflake.epsf scaled 580}\end{center}\end{figure}$

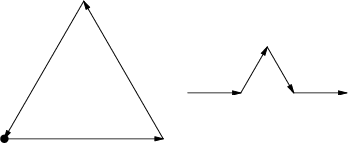
A Fractal derived from the Koch Snowflake. The base curve and motif for the fractal are illustrated below.

$\begin{figure}\begin{center}\BoxedEPSF{KochAntisnowflakeMotif.epsf scaled 800}\end{center}\end{figure}$

The Area after the th iteration is

$\begin{displaymath} A_n = A_{n-1}-{1\over 3}{\ell_{n-1}\over a}{\Delta\over 3^n}, \end{displaymath}$

where $\Delta$ is the area of the original Equilateral Triangle, so from the derivation for the Koch Snowflake,

$\begin{displaymath} A \equiv \lim_{n\to\infty} A_n = (1-{\textstyle{3\over 5}})\Delta = {\textstyle{2\over 5}}\Delta. \end{displaymath}$

References

Cundy, H. and Rollett, A. Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., pp. 66-67, 1989.

Lauwerier, H. Fractals: Endlessly Repeated Geometric Figures. Princeton, NJ: Princeton University Press, pp. 36-37, 1991.

Weisstein, E. W. ``Fractals.'' Mathematica notebook Fractal.m.