|
|
|
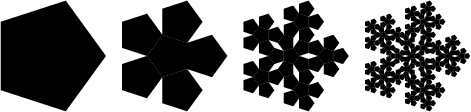
A Fractal with 5-fold symmetry. As illustrated above, five Pentagons can be arranged around an identical Pentagon to form the first iteration of the pentaflake. This cluster of six pentagons has the shape of a pentagon with five triangular wedges removed. This construction was first noticed by Albrecht Dürer (Dixon 1991).
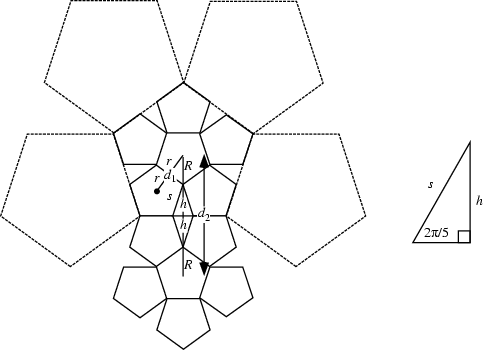
For a pentagon of side length 1, the first ring of pentagons has centers at Radius
| (1) |
| (2) |
| (3) |
| (4) |
| (5) |
| (6) |
Now, the length of the side of the first pentagon compound is given by
| (7) |
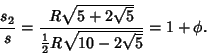 |
(8) |
We can now calculate the dimension of the pentaflake fractal. Let ![]() be the number of black pentagons and
be the number of black pentagons and ![]() the length of
side of a pentagon after the
the length of
side of a pentagon after the ![]() iteration,
iteration,
| (9) | |||
| (10) |
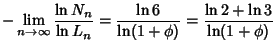 |
|||
| (11) |
See also Pentagon
References
Dixon, R. Mathographics. New York: Dover, pp. 186-188, 1991.
![]() Weisstein, E. W. ``Fractals.'' Mathematica notebook Fractal.m.
Weisstein, E. W. ``Fractals.'' Mathematica notebook Fractal.m.
|
|
|
© 1996-9 Eric W. Weisstein