|
|
|
The number
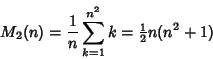
For a Magic Cube, the magic constant is
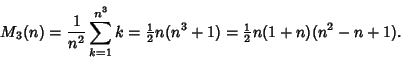
There is a corresponding multiplicative magic constant for Multiplication Magic Squares.
See also Magic Cube, Magic Geometric Constants, Magic Hexagon, Magic Square, Multiplication Magic Square, Panmagic Square
References
Hunter, J. A. H. and Madachy, J. S. ``Mystic Arrays.'' Ch. 3 in Mathematical Diversions. New York: Dover,
pp. 23-34, 1975.
Madachy, J. S. Madachy's Mathematical Recreations. New York: Dover, p. 86, 1979.
Sloane, N. J. A. Sequences
A027441 and
A006003/M3849
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.