|
|
|
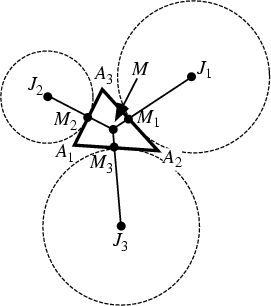
The Lemoine Point of the Excentral Triangle, i.e., the point of concurrence ![]() of the lines from the
Excenters
of the lines from the
Excenters ![]() through the corresponding Triangle side Midpoint
through the corresponding Triangle side Midpoint ![]() . It is also called
the Middlespoint and has Triangle Center Function
. It is also called
the Middlespoint and has Triangle Center Function
See also Excenter, Excentral Triangle, Nagel Point
References
Baptist, P. Die Entwicklung der Neueren Dreiecksgeometrie. Mannheim: Wissenschaftsverlag, p. 72, 1992.
Eddy, R. H. ``A Generalization of Nagel's Middlespoint.'' Elem. Math. 45, 14-18, 1990.
Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994.
Kimberling, C. ``Mittenpunkt.''
http://cedar.evansville.edu/~ck6/tcenters/class/mitten.html.