|
|
|
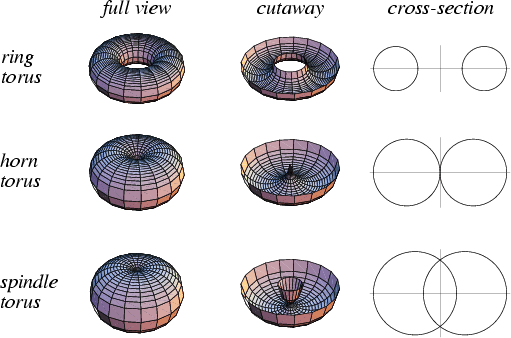
One of the three classes of Tori illustrated above and given by the parametric equations
| (1) | |||
| (2) | |||
| (3) |
The standard tori and their inversions are Cyclides.
See also Apple, Cyclide, Horn Torus, Lemon, Ring Torus, Spindle Torus, Torus
References
Pinkall, U. ``Cyclides of Dupin.'' §3.3 in
Mathematical Models from the Collections of Universities and Museums (Ed. G. Fischer).
Braunschweig, Germany: Vieweg, pp. 28-30, 1986.