|
|
|
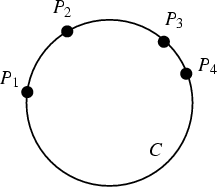
Four or more points ![]() ,
, ![]() ,
, ![]() ,
, ![]() , ... which lie on a Circle
, ... which lie on a Circle ![]() are said to be concyclic. Three
points are trivially concyclic since three noncollinear points determine a Circle. The number of the
are said to be concyclic. Three
points are trivially concyclic since three noncollinear points determine a Circle. The number of the ![]() Lattice Points
Lattice Points ![]() which can be picked with no four concyclic is
which can be picked with no four concyclic is
![]() (Guy 1994).
(Guy 1994).
A theorem states that if any four consecutive points of a Polygon are not concyclic, then its Area can be increased by making them concyclic. This fact arises in some Proofs that the solution to the Isoperimetric Problem is the Circle.
See also Circle, Collinear, Concentric, Cyclic Hexagon, Cyclic Pentagon, Cyclic Quadrilateral, Eccentric, N-Cluster
References
Guy, R. K. ``Lattice Points, No Four on a Circle.'' §F3 in
Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, p. 241, 1994.