|
|
|
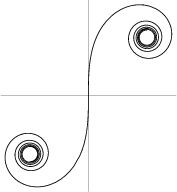
A plot in the Complex Plane of the points
| (1) |
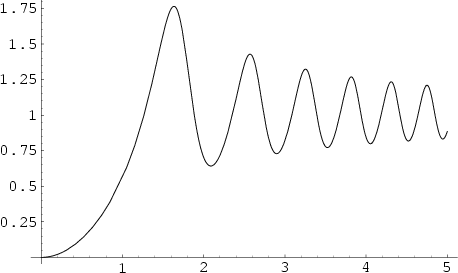
The Slope of the Cornu spiral
| (2) |
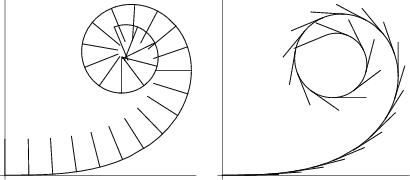
The Slope of the curve's Tangent Vector (above right figure) is
| (3) |
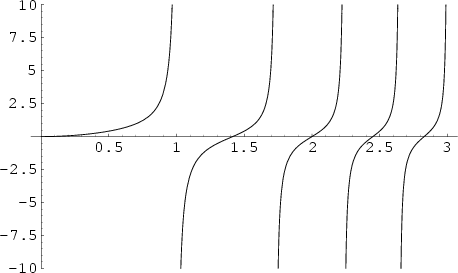
The Cesàro Equation for a Cornu spiral is ![]() , where
, where ![]() is the Radius of
Curvature and
is the Radius of
Curvature and ![]() the Arc Length. The Torsion is
the Arc Length. The Torsion is ![]() .
.
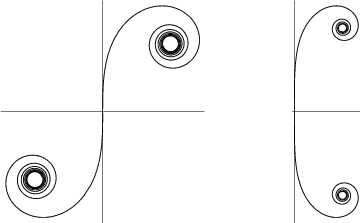
Gray (1993) defines a generalization of the Cornu spiral given by parametric equations
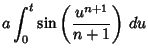 |
(4) | ||
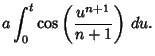 |
(5) |
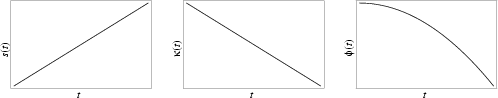
The Arc Length, Curvature, and Tangential Angle of this curve are
| (6) | |||
| (7) | |||
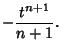 |
(8) |
| (9) |
Dillen (1990) describes a class of ``polynomial spirals'' for which the Curvature is a polynomial function of the Arc Length. These spirals are a further generalization of the Cornu spiral.
See also Fresnel Integrals, Nielsen's Spiral
References
Dillen, F. ``The Classification of Hypersurfaces of a Euclidean Space with Parallel Higher Fundamental Form.''
Math. Z. 203, 635-643, 1990.
Gray, A. ``Clothoids.'' §3.6 in Modern Differential Geometry of Curves and Surfaces.
Boca Raton, FL: CRC Press, pp. 50-52, 1993.
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 190-191, 1972.
|
|
|
© 1996-9 Eric W. Weisstein