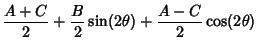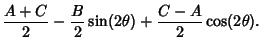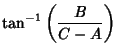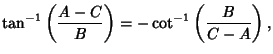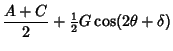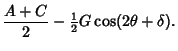Given a general Quadratic Curve
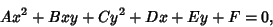 |
(1) |
the quantity 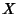 is known as the discriminant, where
is known as the discriminant, where
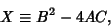 |
(2) |
and is invariant under Rotation. Using the Coefficients from Quadratic
Equations for a rotation by an angle 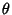 ,
,
Now let
and use
to rewrite the primed variables
From (11) and (13), it follows that
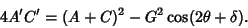 |
(14) |
Combining with (12) yields, for an arbitrary 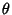
which is therefore invariant under rotation. This invariant therefore provides a useful shortcut to determining the
shape represented by a Quadratic Curve. Choosing 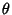 to make
to make  (see Quadratic Equation), the
curve takes on the form
(see Quadratic Equation), the
curve takes on the form
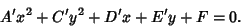 |
(16) |
Completing the Square and defining new variables gives
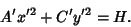 |
(17) |
Without loss of generality, take the sign of 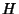 to be positive. The discriminant is
to be positive. The discriminant is
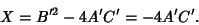 |
(18) |
Now, if 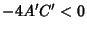 , then
, then 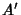 and
and 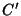 both have the same sign, and the equation has the general form of an
Ellipse (if
both have the same sign, and the equation has the general form of an
Ellipse (if 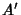 and
and 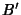 are positive). If
are positive). If 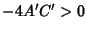 , then
, then 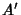 and
and 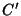 have opposite signs, and the equation has
the general form of a Hyperbola. If
have opposite signs, and the equation has
the general form of a Hyperbola. If 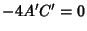 , then either
, then either 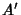 or
or 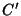 is zero, and the equation has the
general form of a Parabola (if the Nonzero
is zero, and the equation has the
general form of a Parabola (if the Nonzero 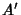 or
or 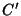 is positive). Since the discriminant is invariant, these
conclusions will also hold for an arbitrary choice of
is positive). Since the discriminant is invariant, these
conclusions will also hold for an arbitrary choice of 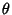 , so they also hold when
, so they also hold when 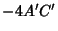 is replaced by the
original
is replaced by the
original 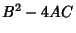 . The general result is
. The general result is
- 1. If
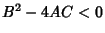 , the equation represents an Ellipse, a Circle (degenerate Ellipse),
a Point (degenerate Circle), or has no graph.
, the equation represents an Ellipse, a Circle (degenerate Ellipse),
a Point (degenerate Circle), or has no graph.
- 2. If
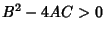 , the equation represents a Hyperbola or pair of intersecting lines (degenerate
Hyperbola).
, the equation represents a Hyperbola or pair of intersecting lines (degenerate
Hyperbola).
- 3. If
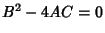 , the equation represents a Parabola, a Line (degenerate Parabola),
a pair of Parallel lines (degenerate Parabola), or has no graph.
, the equation represents a Parabola, a Line (degenerate Parabola),
a pair of Parallel lines (degenerate Parabola), or has no graph.
© 1996-9 Eric W. Weisstein
1999-05-24