Let three similar Isosceles Triangles 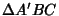 ,
,
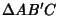 , and
, and 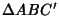 be constructed on the sides of a Triangle
be constructed on the sides of a Triangle
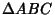 . Then the Envelope of the axis of the Triangles
. Then the Envelope of the axis of the Triangles
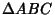 and
and 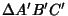 is Kiepert's parabola, given by
is Kiepert's parabola, given by
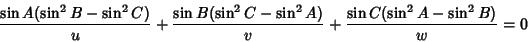 |
(1) |
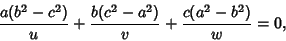 |
(2) |
where ![$[u, v, w]$](k_508.gif) are the Trilinear Coordinates for a line tangent to the parabola. It is tangent to the sides of
the Triangle, the line at infinity, and the Lemoine Line. The Focus has Triangle Center
Function
are the Trilinear Coordinates for a line tangent to the parabola. It is tangent to the sides of
the Triangle, the line at infinity, and the Lemoine Line. The Focus has Triangle Center
Function
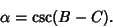 |
(3) |
The Euler Line of a triangle is the Directrix of Kiepert's parabola. In fact, the
Directrices of all parabolas inscribed in a Triangle pass through the
Orthocenter. The Brianchon Point for Kiepert's parabola is the
Steiner Point.
See also Brianchon Point, Envelope, Euler Line, Isosceles Triangle,
Lemoine Line, Steiner Points
© 1996-9 Eric W. Weisstein
1999-05-26
![]() ,
,
![]() , and
, and ![]() be constructed on the sides of a Triangle
be constructed on the sides of a Triangle
![]() . Then the Envelope of the axis of the Triangles
. Then the Envelope of the axis of the Triangles
![]() and
and ![]() is Kiepert's parabola, given by
is Kiepert's parabola, given by