|
|
|
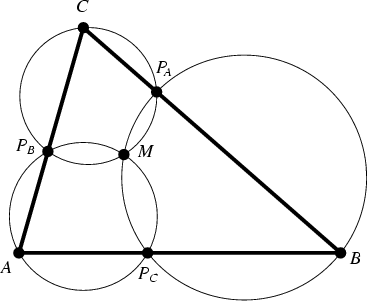
If a point is marked on each side of a Triangle ![]() , then the three Miquel Circles (each through a
Vertex and the two marked points on the adjacent sides) are Concurrent at a point
, then the three Miquel Circles (each through a
Vertex and the two marked points on the adjacent sides) are Concurrent at a point ![]() called
the Miquel Point. This result is a slight generalization of the so-called Pivot Theorem.
called
the Miquel Point. This result is a slight generalization of the so-called Pivot Theorem.
If ![]() lies in the interior of the triangle, then it satisfies
lies in the interior of the triangle, then it satisfies
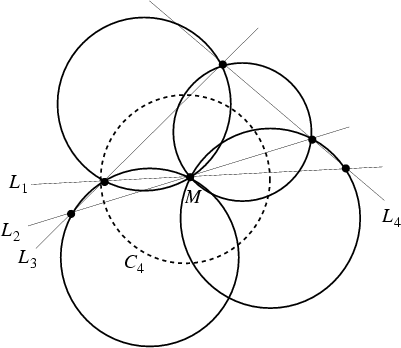
Given four lines ![]() , ...,
, ..., ![]() each intersecting the other three, the four Miquel Circles passing through
each subset of three intersection points of the lines meet in a point known as the 4-Miquel point
each intersecting the other three, the four Miquel Circles passing through
each subset of three intersection points of the lines meet in a point known as the 4-Miquel point ![]() . Furthermore, the
centers of these four Miquel Circles lie on a Circle
. Furthermore, the
centers of these four Miquel Circles lie on a Circle ![]() (Johnson 1929, p. 139). The lines from
(Johnson 1929, p. 139). The lines from ![]() to
given points on the sides make equal Angles with respect to the sides.
to
given points on the sides make equal Angles with respect to the sides.
Similarly, given ![]() lines taken by
lines taken by ![]() s yield
s yield ![]() Miquel Circles like
Miquel Circles like ![]() passing through a point
passing through a point ![]() ,
and their centers lie on a Circle
,
and their centers lie on a Circle ![]() .
.
See also Miquel Circles, Miquel Equation, Miquel Triangle, Nine-Point Circle, Pedal Circle, Pivot Theorem
References
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle.
Boston, MA: Houghton Mifflin, pp. 131-144, 1929.