Nine-Point Circle

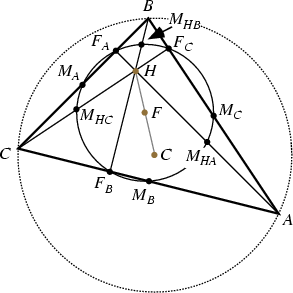
The Circle, also called Euler's Circle and the Feuerbach Circle, which passes through the feet of the Perpendicular $F_A$, $F_B$, and $F_C$ dropped from the Vertices of any Triangle $\Delta ABC$ on the sides opposite them. Euler showed in 1765 that it also passes through the Midpoints $M_A$, $M_B$, $M_C$ of the sides of $\Delta ABC$.

By Feuerbach's Theorem, the nine-point circle also passes through the Midpoints $M_{HA}$, $M_{HB}$, $M_{HC}$ of the segments which join the Vertices and the Orthocenter $H$. These three triples of points make nine in all, giving the circle its name. The center $F$ of the nine-point circle is called the Nine-Point Center.

The Radius of the nine-point circle is $R/2$, where $R$ is the Circumradius. The center of Kiepert's Hyperbola lies on the nine-point circle. The nine-point circle bisects any line from the Orthocenter to a point on the Circumcircle. The nine-point circle of the Incenter and Excenters of a Triangle is the Circumcircle.

The sum of the powers of the Vertices with regard to the nine-point circle is

$${\textstyle{1\over 4}}({a_1}^2+{a_2}^2+{a_3}^2).$$

Also,

$$\overline{FA_1}^2+\overline{FA_2}^2+\overline{FA_3}^2+\overline{FH}^2=3R^2,$$

where $F$ is the Nine-Point Center, $A_i$ are the Vertices, $H$ is the Orthocenter, and $R$ is the Circumradius. All triangles inscribed in a given Circle and having the same Orthocenter have the same nine-point circle.

See also Complete Quadrilateral, Eight-Point Circle Theorem, Feuerbach's Theorem, Fontené Theorems, Griffiths' Theorem, Nine-Point Center, Nine-Point Conic, Orthocentric System

References

Altshiller-Court, N. College Geometry: A Second Course in Plane Geometry for Colleges and Normal Schools, 2nd ed., rev. enl. New York: Barnes and Noble, pp. 93-97, 1952.

Brand, L. ``The Eight-Point Circle and the Nine-Point Circle.'' Amer. Math. Monthly 51, 84-85, 1944.

Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. New York: Random House, pp. 20-22, 1967.

Dörrie, H. ``The Feuerbach Circle.'' §28 in 100 Great Problems of Elementary Mathematics: Their History and Solutions. New York: Dover, pp. 142-144, 1965.

Gardner, M. Mathematical Carnival: A New Round-Up of Tantalizers and Puzzles from Scientific American. New York: Vintage Books, p. 59, 1977.

Guggenbuhl, L. ``Karl Wilhelm Feuerbach, Mathematician.'' Appendix to Circles: A Mathematical View, rev. ed. Washington, DC: Math. Assoc. Amer., pp. 89-100, 1995.

Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 165 and 195-212, 1929.

Lange, J. Geschichte des Feuerbach'schen Kreises. Berlin, 1894.

Mackay, J. S. ``History of the Nine-Point Circle.'' Proc. Edinburgh Math. Soc. 11, 19-61, 1892.

Ogilvy, C. S. Excursions in Geometry. New York: Dover, pp. 119-120, 1990.

Pedoe, D. Circles: A Mathematical View, rev. ed. Washington, DC: Math. Assoc. Amer., pp. 1-4, 1995.