Curves with Cartesian equation
with 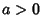 . The above equation represents the third class of Newton's
. The above equation represents the third class of Newton's  classification of
Cubic Curves, which Newton divided into five species depending on the Roots of the
cubic in
classification of
Cubic Curves, which Newton divided into five species depending on the Roots of the
cubic in 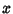 on the right-hand side of the equation. Newton described these cases as having the following characteristics:
on the right-hand side of the equation. Newton described these cases as having the following characteristics:
- 1. ``All the Roots are Real and unequal. Then the Figure is a diverging
Parabola of the Form of a Bell, with an Oval at its Vertex.
- 2. Two of the Roots are equal. A Parabola will be formed, either Nodated by touching an Oval,
or Punctate, by having the Oval infinitely small.
- 3. The three Roots are equal. This is the Neilian Parabola, commonly
called Semi-cubical.
- 4. Only one Real Root. If two of the Roots are impossible, there will be
a Pure Parabola of a Bell-like Form''
(MacTutor Archive).
References
MacTutor History of Mathematics Archive. ``Newton's Diverging Parabolas.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Newtons.html.
© 1996-9 Eric W. Weisstein
1999-05-25