|
|
|
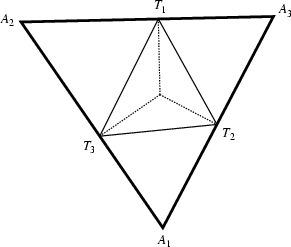
The antipedal triangle ![]() of a given Triangle
of a given Triangle ![]() is the Triangle of which
is the Triangle of which ![]() is the Pedal
Triangle. For a Triangle with Trilinear Coordinates
is the Pedal
Triangle. For a Triangle with Trilinear Coordinates
![]() and Angles
and Angles ![]() ,
,
![]() , and
, and ![]() , the antipedal triangle has Vertices with Trilinear Coordinates
, the antipedal triangle has Vertices with Trilinear Coordinates
See also Pedal Triangle
References
Gallatly, W. The Modern Geometry of the Triangle, 2nd ed. London: Hodgson, pp. 56-58, 1913.