Cone

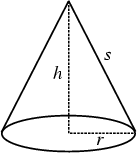
$\begin{figure}\BoxedEPSF{ConeDimensions.epsf}\end{figure}$

A cone is a Pyramid with a circular Cross-Section. A right cone is a cone with its vertex above the center of its base. A right cone of height can be described by the parametric equations

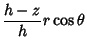$\displaystyle x$	$\textstyle =$	$\displaystyle {h-z\over h}r\cos\theta$	(1)
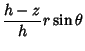$\displaystyle y$	$\textstyle =$	$\displaystyle {h-z\over h}r\sin\theta$	(2)
$\displaystyle z$	$\textstyle =$	$\displaystyle z$	(3)

for $z\in [0,h]$ and $\theta\in [0,2\pi)$ . The Volume of a cone is therefore

$\begin{displaymath} V = {\textstyle{1\over 3}}A_bh, \end{displaymath}$

(4)

where

is the base Area and

is the height. If the base is circular, then

$\begin{displaymath} V={\textstyle{1\over 3}}\pi r^2h. \end{displaymath}$

(5)

This amazing fact was first discovered by Eudoxus,

and other proofs were subsequently found by Archimedes

in On the Sphere and Cylinder (ca. 225 BC

) and Euclid

in Proposition XII.10 of his Elements (Dunham 1990).

The Centroid can be obtained by setting in the equation for the centroid of the Conical Frustum,

$\begin{displaymath} \bar z={\left\langle{z}\right\rangle{}\over V}={h({R_1}^2+R_1R_2+{R_2}^2)\over 4({R_1}^2+2R_1R_2+3{R_2}^2)}, \end{displaymath}$

(6)

(Beyer 1987, p. 133) yielding

$\begin{displaymath} \bar z={\textstyle{1\over 4}}h. \end{displaymath}$

(7)

For a right circular cone, the Slant Height is

$\begin{displaymath} s=\sqrt{r^2+h^2} \end{displaymath}$

(8)

and the surface Area (not including the base) is

$\begin{displaymath} S=\pi rs=\pi r\sqrt{r^2+h^2}. \end{displaymath}$

(9)

In discussions of Conic Sections, the word cone is often used to refer to two similar cones placed apex to apex. This allows the Hyperbola to be defined as the intersection of a Plane with both Nappes (pieces) of the cone.

The Locus of the apex of a variable cone containing an Ellipse fixed in 3-space is a Hyperbola through the Foci of the Ellipse. In addition, the Locus of the apex of a cone containing that Hyperbola is the original Ellipse. Furthermore, the Eccentricities of the Ellipse and Hyperbola are reciprocals.

See also Conic Section, Conical Frustum, Cylinder, Nappe, Pyramid, Sphere

References

Beyer, W. H. (Ed.) CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 129 and 133, 1987.

Dunham, W. Journey Through Genius: The Great Theorems of Mathematics. New York: Wiley, pp. 76-77, 1990.

Yates, R. C. ``Cones.'' A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 34-35, 1952.