|
|
|
Machin-like formulas have the form
| (1) |
Maclaurin-like formulas can be derived by writing
| (2) |
| (3) |
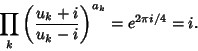 |
(4) |
| (5) |
| (6) |
| (7) |
There are only four such Formulas,
| (8) | |||
| (9) | |||
| (10) | |||
| (11) |
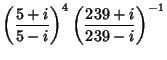 |
(12) | ||
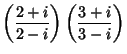 |
(13) | ||
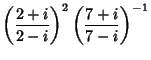 |
(14) | ||
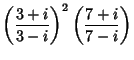 |
(15) |
Machin-like formulas with two terms can also be generated which do not have integral arc cotangent arguments such as
Euler's ![]()
| (16) |
| (17) |
Three-term Machin-like formulas include Gauss's Machin-Like Formula
| (18) |
| (19) |
| (20) | |||
| (21) | |||
| (22) | |||
| (23) | |||
| (24) |
Using trigonometric identities such as
| (25) |
The efficiency of a Formula is the time it takes to calculate ![]() with the Power series for arctangent
with the Power series for arctangent
| (26) |
| (27) |
|
|
|
|
|
(28) |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
(29) |
| (30) |
The following table gives the number ![]() of Machin-like formulas of
of Machin-like formulas of ![]() terms in the compilation by Wetherfield and
Hwang. Except for previously known identities (which are included), the criteria for inclusion are the following:
terms in the compilation by Wetherfield and
Hwang. Except for previously known identities (which are included), the criteria for inclusion are the following:
| 1 | 1 | 0 |
| 2 | 4 | 1.85113 |
| 3 | 106 | 1.78661 |
| 4 | 39 | 1.58604 |
| 5 | 90 | 1.63485 |
| 6 | 120 | 1.51244 |
| 7 | 113 | 1.54408 |
| 8 | 18 | 1.65089 |
| 9 | 4 | 1.72801 |
| 10 | 78 | 1.63086 |
| 11 | 34 | 1.6305 |
| 12 | 188 | 1.67458 |
| 13 | 37 | 1.71934 |
| 14 | 5 | 1.75161 |
| 15 | 24 | 1.77957 |
| 16 | 51 | 1.81522 |
| 17 | 5 | 1.90938 |
| 18 | 570 | 1.87698 |
| 19 | 1 | 1.94899 |
| 20 | 11 | 1.95716 |
| 21 | 1 | 1.98938 |
| Total | 1500 | 1.51244 |
See also Euler's Machin-Like Formula, Gauss's Machin-Like Formula, Gregory Number, Hermann's Formula, Hutton's Formula, Inverse Cotangent, Machin's Formula, Pi, Størmer Number, Strassnitzky's Formula
References
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York:
Dover, pp. 347-359, 1987.
Borwein, J. M. and Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity.
New York: Wiley, 1987.
Castellanos, D. ``The Ubiquitous Pi. Part I.'' Math. Mag. 61, 67-98, 1988.
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 241-248, 1996.
Hwang, C.-L. ``More Machin-Type Identities.'' Math. Gaz., 120-121, March 1997.
Lehmer, D. H. ``On Arccotangent Relations for
Lewin, L. Polylogarithms and Associated Functions. New York: North-Holland, 1981.
Lewin, L. Structural Properties of Polylogarithms. Providence, RI: Amer. Math. Soc., 1991.
Nielsen, N. Der Euler'sche Dilogarithms. Leipzig, Germany: Halle, 1909.
Størmer, C. ``Sur l'Application de la Théorie des Nombres Entiers Complexes à la Solution en
Nombres Rationels
Todd, J. ``A Problem on Arc Tangent Relations.'' Amer. Math. Monthly 56, 517-528, 1949.
Wetherfield, M. ``The Enhancement of Machin's Formula by Todd's Process.'' Math. Gaz. 80, 333-344, 1996.
Wetherfield, M. ``Machin Revisited.'' Math. Gaz., 121-123, March 1997.
![]() .'' Amer. Math. Monthly 45, 657-664, 1938.
.'' Amer. Math. Monthly 45, 657-664, 1938.
![]() ,
, ![]() , ...,
, ..., ![]() ,
, ![]() , ...,
, ..., ![]() de l'Equation....''
Archiv for Mathematik og Naturvidenskab B 19, 75-85, 1896.
de l'Equation....''
Archiv for Mathematik og Naturvidenskab B 19, 75-85, 1896.
![]() Weisstein, E. W. ``Machin-Like Formulas.'' Mathematica notebook MachinFormulas.m.
Weisstein, E. W. ``Machin-Like Formulas.'' Mathematica notebook MachinFormulas.m.
|
|
|
© 1996-9 Eric W. Weisstein