|
|
|
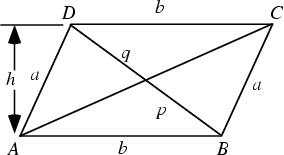
A Quadrilateral with opposite sides parallel (and therefore opposite angles equal). A quadrilateral with equal sides is called a Rhombus, and a parallelogram whose Angles are all Right Angles is called a Rectangle.
A parallelogram of base ![]() and height
and height ![]() has Area
has Area
| (1) |
| (2) |
| (3) | |||
| (4) | |||
| (5) |
The Area of the parallelogram with sides formed by the Vectors ![]() and
and ![]() is
is
| (6) |
| (7) |
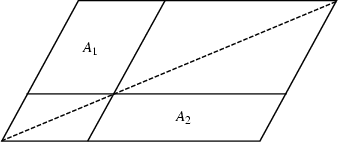
As shown by Euclid, ![]() if lines parallel to the sides are drawn through any point on a diagonal of a
parallelogram, then the parallelograms not containing segments of that diagonal are equal in Area (and conversely), so
in the above figure,
if lines parallel to the sides are drawn through any point on a diagonal of a
parallelogram, then the parallelograms not containing segments of that diagonal are equal in Area (and conversely), so
in the above figure, ![]() (Johnson 1929).
(Johnson 1929).
See also Diamond, Lozenge, Parallelogram Illusion, Rectangle, Rhombus, Varignon Parallelogram, Wittenbauer's Parallelogram
References
Beyer, W. H. (Ed.) CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 123, 1987.
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA:
Houghton Mifflin, p. 61, 1929.
|
|
|
© 1996-9 Eric W. Weisstein