|
|
|
A Non-Euclidean Geometry, also called Lobachevsky-Bolyai-Gauss Geometry, having constant Sectional Curvature ![]() . This Geometry satisfies all of Euclid's
Postulates except the Parallel Postulate, which is modified to read: For any infinite straight Line
. This Geometry satisfies all of Euclid's
Postulates except the Parallel Postulate, which is modified to read: For any infinite straight Line
![]() and any Point
and any Point ![]() not on it, there are many other infinitely extending straight Lines that
pass through
not on it, there are many other infinitely extending straight Lines that
pass through ![]() and which do not intersect
and which do not intersect ![]() .
.
In hyperbolic geometry, the sum of Angles of a Triangle is less than 180°, and Triangles with the same angles have the same areas. Furthermore, not all Triangles have the same Angle sum (c.f. the AAA Theorem for Triangles in Euclidean 2-space). The best-known example of a hyperbolic space are Spheres in Lorentzian 4-space. The Poincaré Hyperbolic Disk is a hyperbolic 2-space. Hyperbolic geometry is well understood in 2-D, but not in 3-D.
Geometric models of hyperbolic geometry include the Klein-Beltrami Model, which consists of an Open Disk in
the Euclidean plane whose open chords correspond to hyperbolic lines. A 2-D model is the Poincaré Hyperbolic
Disk. Felix Klein ![]() constructed an analytic hyperbolic geometry in 1870
in which a Point is represented by a pair of Real Numbers
constructed an analytic hyperbolic geometry in 1870
in which a Point is represented by a pair of Real Numbers ![]() with
with
![\begin{displaymath}
d(x,X) = a\cosh^{-1}\!\!\left[{1-x_1X_1-x_2X_2\over \sqrt{1-{x_1}^2-{x_2}^2}\,\sqrt{1-{X_1}^2-{X_2}^2}}\right]\!\!.
\end{displaymath}](h_2078.gif)
 |
|||
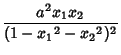 |
|||
 |
See also Elliptic Geometry, Euclidean Geometry, Hyperbolic Metric, Klein-Beltrami Model, Non-Euclidean Geometry, Schwarz-Pick Lemma
References
Dunham, W. Journey Through Genius: The Great Theorems of Mathematics. New York: Wiley, pp. 57-60, 1990.
Eppstein, D. ``Hyperbolic Geometry.''
http://www.ics.uci.edu/~eppstein/junkyard/hyper.html.
Stillwell, J. Sources of Hyperbolic Geometry. Providence, RI: Amer. Math. Soc., 1996.
|
|
|
© 1996-9 Eric W. Weisstein