|
|
|
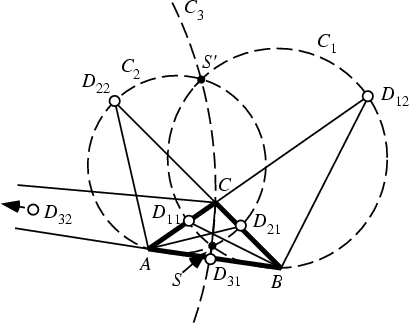
The first and second isodynamic points of a Triangle ![]() can be constructed by drawing the triangle's Angle
Bisectors and Exterior Angle Bisectors. Each pair of bisectors intersects a
side of the triangle (or its extension) in two points
can be constructed by drawing the triangle's Angle
Bisectors and Exterior Angle Bisectors. Each pair of bisectors intersects a
side of the triangle (or its extension) in two points ![]() and
and ![]() , for
, for ![]() , 2, 3. The three Circles having
, 2, 3. The three Circles having
![]() ,
, ![]() , and
, and ![]() as Diameters are the Apollonius Circles
as Diameters are the Apollonius Circles ![]() ,
,
![]() , and
, and ![]() . The points
. The points ![]() and
and ![]() in which the three Apollonius Circles intersect are the first and second
isodynamic points, respectively.
in which the three Apollonius Circles intersect are the first and second
isodynamic points, respectively.
![]() and
and ![]() have Triangle Center Functions
have Triangle Center Functions
The isodynamic points are Isogonal Conjugates of the Isogonic Centers. They lie on the Brocard Axis. The distances from either isodynamic point to the Vertices are inversely proportional to the sides. The Pedal Triangle of either isodynamic point is an Equilateral Triangle. An Inversion with either isodynamic point as the Inversion Center transforms the triangle into an Equilateral Triangle.
The Circle which passes through both the isodynamic points and the Centroid of a Triangle is known as the Parry Circle.
See also Apollonius Circles, Brocard Axis, Centroid (Triangle), Isogonic Centers, Parry Circle
References
Gallatly, W. The Modern Geometry of the Triangle, 2nd ed. London: Hodgson, p. 106, 1913.
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA:
Houghton Mifflin, pp. 295-297, 1929.
Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994.
|
|
|
© 1996-9 Eric W. Weisstein