|
|
|
For a Quadrilateral with sides of length ![]() ,
, ![]() ,
, ![]() , and
, and ![]() , the Area
, the Area ![]() is given by
is given by
| (1) |
| (2) |
| (3) | |||
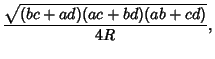 |
(4) |
| (5) |
See also Bretschneider's Formula, Heron's Formula
References
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 56-60,
1967.
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA:
Houghton Mifflin, pp. 81-82, 1929.